Home > Topdrawer > Patterns > Misunderstandings > Number sequences > Let's have a party!
Let's have a party!
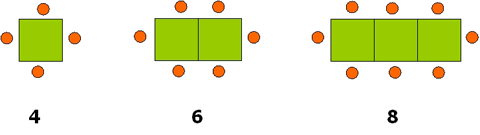
Roma is having a party. She wants her guests to sit at a row of square tables. She knows that she can seat one person on each side of a table, but she does not know how to work out how many tables she will need. Can your students help her?
Students may suggest making drawings of the tables for some possible numbers of guests. If so, let them struggle with this task for a little while and then suggest that, instead, they make drawings for various numbers of tables.
After some experimentation, ask students how they could organise their data better. One possibility is to represent their results as a growing pattern.
Numbers of guests for various numbers of tables.
Now they can see that the maximum number of guests that can be seated increases by two for each additional table.
Why two? Because two of the four possible positions around each table are eliminated when two tables are placed next to each other.
So how do you work out the number of tables Roma needs from the number of guests?
After students have puzzled over this question, look at this discussion of Roma's Party.
